Unveiling the History of the Finite Element Method
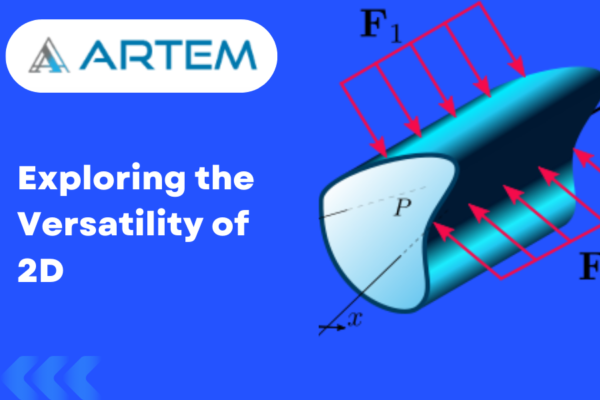
A finite element method (FEM) is a numerical method used for solving engineering and mathematical problems involving the distribution of complex systems or structures into smaller, simpler, and interconnected subdomains. A set of mathematical equations approximates the behavior of each element. It has been widely applied in many fields, including structural analysis, heat transfer, fluid dynamics, and electromagnetics.
The history of the Finite Element Method dates back to the early 1940s, with the work of Richard Courant, a German mathematician. Courant, along with his collaborators, developed a numerical technique called the Ritz-Galerkin method for approximating solutions to differential equations. This method laid the foundation for what would later become the Finite Element Method.

In the late 1950s, engineers and mathematicians began developing the concept of dividing structures into small subdomains to simplify analysis. Notable pioneers in the development of FEM during this time include J.H. Argyris, Ray W. Clough, and Olek Zienkiewicz. They published seminal papers outlining the mathematical foundations and practical applications of the method.
The first recorded use of the term “finite element” in the context of structural analysis dates back to the 1960s. It was coined by two engineers, Ray W. Clough and G. Temple, in their 1965 paper titled “The Finite Element Method in Plane Stress Analysis.”
In this paper, Clough and Temple described their approach to solving plane stress analysis problems using a numerical method they referred to as the “finite element method.” They introduced the concept of dividing the domain into small subdomains (finite elements) and deriving the governing equations for each element. The authors highlighted the benefits of this method, including its ability to handle irregular geometries and complex boundary conditions.
Since then, the term “finite element” has become the widely accepted terminology for this numerical technique, and it has been used consistently in subsequent research papers, books, and software development related to the method.
The first book on the finite element method was “The Mathematical Foundations of the Finite Element Method” by Jacques Louis Lions and Olgierd C. Zienkiewicz. It was published in 1972. This book provided a comprehensive mathematical treatment of the finite element method, outlining the underlying principles and mathematical formulations involved in solving problems using the method. It became a seminal reference for researchers and practitioners in the field and played a significant role in the popularization and advancement of the finite element method.

In 1960, Zienkiewicz, a British engineer, published a landmark paper titled “The Finite Element Method in Structural and Continuum Mechanics,” which introduced the term “finite element method” and presented the formulation of the method for structural analysis. Zienkiewicz’s work helped popularize the method and laid the groundwork for its subsequent development and application in various fields.
The first finite element software was developed by a team led by Richard H. Gallagher at the Structural Analysis Group at the University of California, Berkeley. The software, known as STRESS, was created in the early 1960s and was primarily used for structural analysis.

STRESS was one of the earliest implementations of the finite element method and was initially developed for linear elasticity problems. It allowed engineers to input the geometry, material properties, and boundary conditions of a structure and then perform stress analysis calculations using the finite element method. The software utilized the matrix displacement method, which is a precursor to the more widely used displacement-based formulation.
While STRESS was significant in pioneering the application of the finite element method, it was a relatively simple program compared to modern finite element software packages. Over the years, the development of commercial software such as NASTRAN, ABAQUS, ANSYS, and many others has greatly expanded the capabilities and applications of the finite element method.

Expansion and Diversification: Throughout the 1970s and 1980s, the finite element method expanded rapidly into various fields of engineering and science. Researchers extended its applications to areas such as heat transfer, fluid dynamics, and electromagnetics. The method’s flexibility and ability to handle complex geometries and boundary conditions contributed to its popularity.
Advancements and Refinements: Over the years, researchers have continually refined and enhanced the finite element method.
The development of powerful computers in the latter half of the 20th century greatly accelerated the progress of the Finite Element Method. The availability of computational resources enabled engineers and scientists to solve more complex problems and perform more accurate simulations. Commercial software packages dedicated to finite
element analysis (FEA) emerged, making the method more accessible and practical for engineering applications.
Since its inception, the Finite Element Method has continued to evolve, with improvements in element formulations, numerical algorithms, and computer hardware. It has become a standard tool in engineering and scientific communities, offering efficient and accurate solutions for a wide range of problems involving structural, thermal, fluid, and electromagnetic analyses.
Today, the Finite Element Method remains a prominent and indispensable numerical technique, widely used in industries such as aerospace, automotive, civil engineering, and biomechanics, among others. Its versatility and robustness have made it a cornerstone of modern computational engineering and analysis.